Welcome to Subscribe On Youtube
1334. Find the City With the Smallest Number of Neighbors at a Threshold Distance
Description
There are n cities numbered from 0 to n-1. Given the array edges where edges[i] = [fromi, toi, weighti] represents a bidirectional and weighted edge between cities fromi and toi, and given the integer distanceThreshold.
Return the city with the smallest number of cities that are reachable through some path and whose distance is at most distanceThreshold, If there are multiple such cities, return the city with the greatest number.
Notice that the distance of a path connecting cities i and j is equal to the sum of the edges' weights along that path.
Example 1:
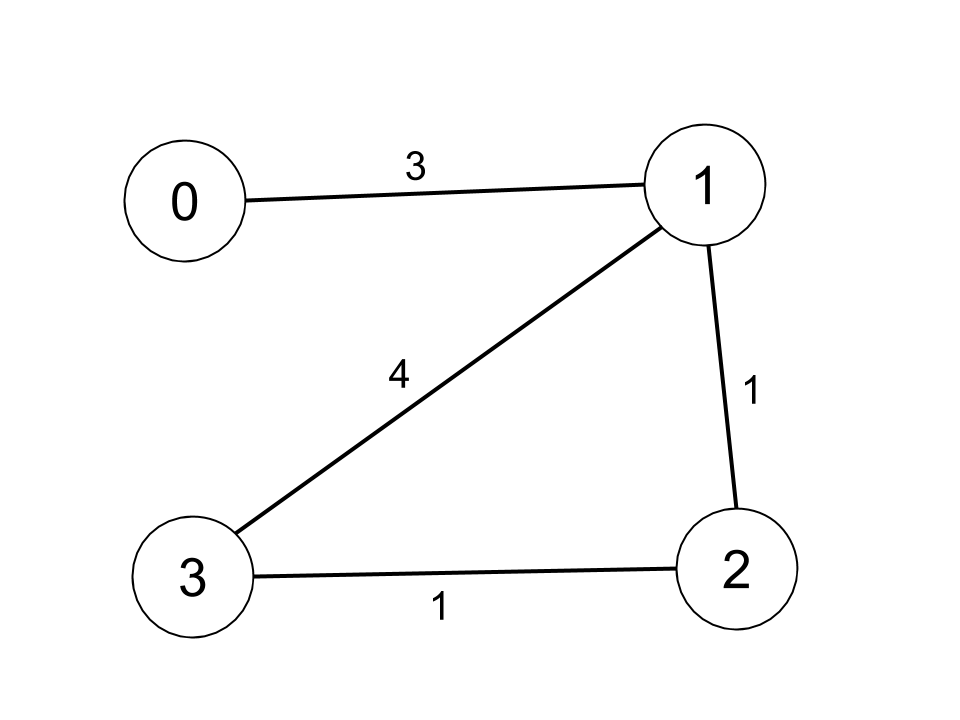
Input: n = 4, edges = [[0,1,3],[1,2,1],[1,3,4],[2,3,1]], distanceThreshold = 4 Output: 3 Explanation: The figure above describes the graph. The neighboring cities at a distanceThreshold = 4 for each city are: City 0 -> [City 1, City 2] City 1 -> [City 0, City 2, City 3] City 2 -> [City 0, City 1, City 3] City 3 -> [City 1, City 2] Cities 0 and 3 have 2 neighboring cities at a distanceThreshold = 4, but we have to return city 3 since it has the greatest number.
Example 2:
Input: n = 5, edges = [[0,1,2],[0,4,8],[1,2,3],[1,4,2],[2,3,1],[3,4,1]], distanceThreshold = 2 Output: 0 Explanation: The figure above describes the graph. The neighboring cities at a distanceThreshold = 2 for each city are: City 0 -> [City 1] City 1 -> [City 0, City 4] City 2 -> [City 3, City 4] City 3 -> [City 2, City 4] City 4 -> [City 1, City 2, City 3] The city 0 has 1 neighboring city at a distanceThreshold = 2.
Constraints:
2 <= n <= 1001 <= edges.length <= n * (n - 1) / 2edges[i].length == 30 <= fromi < toi < n1 <= weighti, distanceThreshold <= 10^4- All pairs
(fromi, toi)are distinct.
Solutions
Solution 1: Dijkstra’S Algorithm
We can enumerate each city $i$ as the starting point, use Dijkstra’s algorithm to find the shortest distance from $i$ to other cities, then count the number of cities whose distance does not exceed the threshold, and finally take the smallest number and the largest numbered city.
Time complexity $O(n^3)$, space complexity $O(n^2)$. Among them, $n$ is the number of cities.
Solution 2
We define $g[i][j]$ to represent the shortest distance from city $i$ to city $j$, initially $g[i][j] = \infty$, $g[i][i] = 0$, then we traverse all edges, for each edge $(f, t, w)$, we let $g[f][t] = g[t][f] = w$.
Next, we use Floyd’s algorithm to find the shortest distance between any two points. Specifically, we first enumerate the intermediate point $k$, then enumerate the upper point $i$ and the end point $j$. If $g[i][k] + g[k][j] \lt g[i][j]$, then we update $g[i][j]$ with the shorter distance $g[i][k] + g[k][j]$.
Finally, we enumerate each city $i$ as the starting point, count the number of cities whose distance does not exceed the threshold, and finally take the smallest number and the largest numbered city.
Time complexity $O(n^3)$, space complexity $O(n^2)$. Among them, $n$ is the number of cities.
-
class Solution { private int n; private int[][] g; private int[] dist; private boolean[] vis; private final int inf = 1 << 30; private int distanceThreshold; public int findTheCity(int n, int[][] edges, int distanceThreshold) { this.n = n; this.distanceThreshold = distanceThreshold; g = new int[n][n]; dist = new int[n]; vis = new boolean[n]; for (var e : g) { Arrays.fill(e, inf); } for (var e : edges) { int f = e[0], t = e[1], w = e[2]; g[f][t] = w; g[t][f] = w; } int ans = n, cnt = inf; for (int i = n - 1; i >= 0; --i) { int t = dijkstra(i); if (t < cnt) { cnt = t; ans = i; } } return ans; } private int dijkstra(int u) { Arrays.fill(dist, inf); Arrays.fill(vis, false); dist[u] = 0; for (int i = 0; i < n; ++i) { int k = -1; for (int j = 0; j < n; ++j) { if (!vis[j] && (k == -1 || dist[k] > dist[j])) { k = j; } } vis[k] = true; for (int j = 0; j < n; ++j) { dist[j] = Math.min(dist[j], dist[k] + g[k][j]); } } int cnt = 0; for (int d : dist) { if (d <= distanceThreshold) { ++cnt; } } return cnt; } } // Solution 2 class Solution { public int findTheCity(int n, int[][] edges, int distanceThreshold) { final int inf = 1 << 29; int[][] g = new int[n][n]; for (var e : g) { Arrays.fill(e, inf); } for (var e : edges) { int f = e[0], t = e[1], w = e[2]; g[f][t] = w; g[t][f] = w; } for (int k = 0; k < n; ++k) { g[k][k] = 0; for (int i = 0; i < n; ++i) { for (int j = 0; j < n; ++j) { g[i][j] = Math.min(g[i][j], g[i][k] + g[k][j]); } } } int ans = n, cnt = inf; for (int i = n - 1; i >= 0; --i) { int t = 0; for (int d : g[i]) { if (d <= distanceThreshold) { ++t; } } if (t < cnt) { cnt = t; ans = i; } } return ans; } } -
class Solution { public: int findTheCity(int n, vector<vector<int>>& edges, int distanceThreshold) { int g[n][n]; int dist[n]; bool vis[n]; memset(g, 0x3f, sizeof(g)); for (auto& e : edges) { int f = e[0], t = e[1], w = e[2]; g[f][t] = g[t][f] = w; } auto dijkstra = [&](int u) { memset(dist, 0x3f, sizeof(dist)); memset(vis, 0, sizeof(vis)); dist[u] = 0; for (int i = 0; i < n; ++i) { int k = -1; for (int j = 0; j < n; ++j) { if (!vis[j] && (k == -1 || dist[j] < dist[k])) { k = j; } } vis[k] = true; for (int j = 0; j < n; ++j) { dist[j] = min(dist[j], dist[k] + g[k][j]); } } return count_if(dist, dist + n, [&](int d) { return d <= distanceThreshold; }); }; int ans = n, cnt = n + 1; for (int i = n - 1; ~i; --i) { int t = dijkstra(i); if (t < cnt) { cnt = t; ans = i; } } return ans; } }; // Solution 2 class Solution { public: int findTheCity(int n, vector<vector<int>>& edges, int distanceThreshold) { int g[n][n]; memset(g, 0x3f, sizeof(g)); for (auto& e : edges) { int f = e[0], t = e[1], w = e[2]; g[f][t] = g[t][f] = w; } for (int k = 0; k < n; ++k) { g[k][k] = 0; for (int i = 0; i < n; ++i) { for (int j = 0; j < n; ++j) { g[i][j] = min(g[i][j], g[i][k] + g[k][j]); } } } int ans = n, cnt = n + 1; for (int i = n - 1; ~i; --i) { int t = count_if(g[i], g[i] + n, [&](int x) { return x <= distanceThreshold; }); if (t < cnt) { cnt = t; ans = i; } } return ans; } }; -
class Solution: def findTheCity( self, n: int, edges: List[List[int]], distanceThreshold: int ) -> int: def dijkstra(u: int) -> int: dist = [inf] * n dist[u] = 0 vis = [False] * n for _ in range(n): k = -1 for j in range(n): if not vis[j] and (k == -1 or dist[k] > dist[j]): k = j vis[k] = True for j in range(n): # dist[j] = min(dist[j], dist[k] + g[k][j]) if dist[k] + g[k][j] < dist[j]: dist[j] = dist[k] + g[k][j] return sum(d <= distanceThreshold for d in dist) g = [[inf] * n for _ in range(n)] for f, t, w in edges: g[f][t] = g[t][f] = w ans, cnt = n, inf for i in range(n - 1, -1, -1): if (t := dijkstra(i)) < cnt: cnt, ans = t, i return ans # Solution 2 class Solution: def findTheCity( self, n: int, edges: List[List[int]], distanceThreshold: int ) -> int: g = [[inf] * n for _ in range(n)] for f, t, w in edges: g[f][t] = g[t][f] = w for k in range(n): g[k][k] = 0 for i in range(n): for j in range(n): # g[i][j] = min(g[i][j], g[i][k] + g[k][j]) if g[i][k] + g[k][j] < g[i][j]: g[i][j] = g[i][k] + g[k][j] ans, cnt = n, inf for i in range(n - 1, -1, -1): t = sum(d <= distanceThreshold for d in g[i]) if t < cnt: cnt, ans = t, i return ans -
func findTheCity(n int, edges [][]int, distanceThreshold int) int { g := make([][]int, n) dist := make([]int, n) vis := make([]bool, n) const inf int = 1e7 for i := range g { g[i] = make([]int, n) for j := range g[i] { g[i][j] = inf } } for _, e := range edges { f, t, w := e[0], e[1], e[2] g[f][t], g[t][f] = w, w } dijkstra := func(u int) (cnt int) { for i := range vis { vis[i] = false dist[i] = inf } dist[u] = 0 for i := 0; i < n; i++ { k := -1 for j := 0; j < n; j++ { if !vis[j] && (k == -1 || dist[j] < dist[k]) { k = j } } vis[k] = true for j := 0; j < n; j++ { dist[j] = min(dist[j], dist[k]+g[k][j]) } } for _, d := range dist { if d <= distanceThreshold { cnt++ } } return } ans, cnt := n, inf for i := n - 1; i >= 0; i-- { if t := dijkstra(i); t < cnt { cnt = t ans = i } } return ans } // Solution 2 func findTheCity(n int, edges [][]int, distanceThreshold int) int { g := make([][]int, n) const inf int = 1e7 for i := range g { g[i] = make([]int, n) for j := range g[i] { g[i][j] = inf } } for _, e := range edges { f, t, w := e[0], e[1], e[2] g[f][t], g[t][f] = w, w } for k := 0; k < n; k++ { g[k][k] = 0 for i := 0; i < n; i++ { for j := 0; j < n; j++ { g[i][j] = min(g[i][j], g[i][k]+g[k][j]) } } } ans, cnt := n, n+1 for i := n - 1; i >= 0; i-- { t := 0 for _, x := range g[i] { if x <= distanceThreshold { t++ } } if t < cnt { cnt, ans = t, i } } return ans } -
function findTheCity(n: number, edges: number[][], distanceThreshold: number): number { const g: number[][] = Array.from({ length: n }, () => Array(n).fill(Infinity)); const dist: number[] = Array(n).fill(Infinity); const vis: boolean[] = Array(n).fill(false); for (const [f, t, w] of edges) { g[f][t] = g[t][f] = w; } const dijkstra = (u: number): number => { dist.fill(Infinity); vis.fill(false); dist[u] = 0; for (let i = 0; i < n; ++i) { let k = -1; for (let j = 0; j < n; ++j) { if (!vis[j] && (k === -1 || dist[j] < dist[k])) { k = j; } } vis[k] = true; for (let j = 0; j < n; ++j) { dist[j] = Math.min(dist[j], dist[k] + g[k][j]); } } return dist.filter(d => d <= distanceThreshold).length; }; let ans = n; let cnt = Infinity; for (let i = n - 1; i >= 0; --i) { const t = dijkstra(i); if (t < cnt) { cnt = t; ans = i; } } return ans; } // Solution 2 function findTheCity(n: number, edges: number[][], distanceThreshold: number): number { const g: number[][] = Array.from({ length: n }, () => Array(n).fill(Infinity)); for (const [f, t, w] of edges) { g[f][t] = g[t][f] = w; } for (let k = 0; k < n; ++k) { g[k][k] = 0; for (let i = 0; i < n; ++i) { for (let j = 0; j < n; ++j) { g[i][j] = Math.min(g[i][j], g[i][k] + g[k][j]); } } } let ans = n, cnt = n + 1; for (let i = n - 1; i >= 0; --i) { const t = g[i].filter(x => x <= distanceThreshold).length; if (t < cnt) { cnt = t; ans = i; } } return ans; } -
function findTheCity(n, edges, distanceThreshold) { const g = Array.from({ length: n }, () => Array(n).fill(Infinity)); const dist = Array(n).fill(Infinity); const vis = Array(n).fill(false); for (const [f, t, w] of edges) { g[f][t] = g[t][f] = w; } const dijkstra = u => { dist.fill(Infinity); vis.fill(false); dist[u] = 0; for (let i = 0; i < n; ++i) { let k = -1; for (let j = 0; j < n; ++j) { if (!vis[j] && (k === -1 || dist[j] < dist[k])) { k = j; } } vis[k] = true; for (let j = 0; j < n; ++j) { dist[j] = Math.min(dist[j], dist[k] + g[k][j]); } } return dist.filter(d => d <= distanceThreshold).length; }; let ans = n; let cnt = Infinity; for (let i = n - 1; i >= 0; --i) { const t = dijkstra(i); if (t < cnt) { cnt = t; ans = i; } } return ans; } // Solution 2 function findTheCity(n, edges, distanceThreshold) { const g = Array.from({ length: n }, () => Array(n).fill(Infinity)); for (const [f, t, w] of edges) { g[f][t] = g[t][f] = w; } for (let k = 0; k < n; ++k) { g[k][k] = 0; for (let i = 0; i < n; ++i) { for (let j = 0; j < n; ++j) { g[i][j] = Math.min(g[i][j], g[i][k] + g[k][j]); } } } let ans = n, cnt = n + 1; for (let i = n - 1; i >= 0; --i) { const t = g[i].filter(x => x <= distanceThreshold).length; if (t < cnt) { cnt = t; ans = i; } } return ans; }