Welcome to Subscribe On Youtube
655. Print Binary Tree
Description
Given the root of a binary tree, construct a 0-indexed m x n string matrix res that represents a formatted layout of the tree. The formatted layout matrix should be constructed using the following rules:
- The height of the tree is
heightand the number of rowsmshould be equal toheight + 1. - The number of columns
nshould be equal to2height+1 - 1. - Place the root node in the middle of the top row (more formally, at location
res[0][(n-1)/2]). - For each node that has been placed in the matrix at position
res[r][c], place its left child atres[r+1][c-2height-r-1]and its right child atres[r+1][c+2height-r-1]. - Continue this process until all the nodes in the tree have been placed.
- Any empty cells should contain the empty string
"".
Return the constructed matrix res.
Example 1:
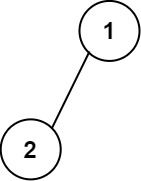
Input: root = [1,2] Output: [["","1",""], ["2","",""]]
Example 2:
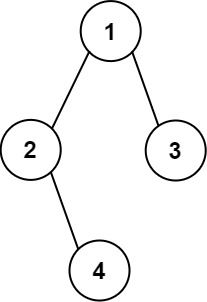
Input: root = [1,2,3,null,4] Output: [["","","","1","","",""], ["","2","","","","3",""], ["","","4","","","",""]]
Constraints:
- The number of nodes in the tree is in the range
[1, 210]. -99 <= Node.val <= 99- The depth of the tree will be in the range
[1, 10].
Solutions
-
/** * Definition for a binary tree node. * public class TreeNode { * int val; * TreeNode left; * TreeNode right; * TreeNode() {} * TreeNode(int val) { this.val = val; } * TreeNode(int val, TreeNode left, TreeNode right) { * this.val = val; * this.left = left; * this.right = right; * } * } */ class Solution { public List<List<String>> printTree(TreeNode root) { int h = height(root); int m = h + 1, n = (1 << (h + 1)) - 1; String[][] res = new String[m][n]; for (int i = 0; i < m; ++i) { Arrays.fill(res[i], ""); } dfs(root, res, h, 0, (n - 1) / 2); List<List<String>> ans = new ArrayList<>(); for (String[] t : res) { ans.add(Arrays.asList(t)); } return ans; } private void dfs(TreeNode root, String[][] res, int h, int r, int c) { if (root == null) { return; } res[r][c] = String.valueOf(root.val); dfs(root.left, res, h, r + 1, c - (1 << (h - r - 1))); dfs(root.right, res, h, r + 1, c + (1 << (h - r - 1))); } private int height(TreeNode root) { if (root == null) { return -1; } return 1 + Math.max(height(root.left), height(root.right)); } } -
/** * Definition for a binary tree node. * struct TreeNode { * int val; * TreeNode *left; * TreeNode *right; * TreeNode() : val(0), left(nullptr), right(nullptr) {} * TreeNode(int x) : val(x), left(nullptr), right(nullptr) {} * TreeNode(int x, TreeNode *left, TreeNode *right) : val(x), left(left), right(right) {} * }; */ class Solution { public: vector<vector<string>> printTree(TreeNode* root) { int h = height(root); int m = h + 1, n = (1 << (h + 1)) - 1; vector<vector<string>> ans(m, vector<string>(n, "")); dfs(root, ans, h, 0, (n - 1) / 2); return ans; } void dfs(TreeNode* root, vector<vector<string>>& ans, int h, int r, int c) { if (!root) return; ans[r][c] = to_string(root->val); dfs(root->left, ans, h, r + 1, c - pow(2, h - r - 1)); dfs(root->right, ans, h, r + 1, c + pow(2, h - r - 1)); } int height(TreeNode* root) { if (!root) return -1; return 1 + max(height(root->left), height(root->right)); } }; -
# Definition for a binary tree node. # class TreeNode: # def __init__(self, val=0, left=None, right=None): # self.val = val # self.left = left # self.right = right class Solution: def printTree(self, root: Optional[TreeNode]) -> List[List[str]]: def height(root): if root is None: return -1 return 1 + max(height(root.left), height(root.right)) def dfs(root, r, c): if root is None: return ans[r][c] = str(root.val) dfs(root.left, r + 1, c - 2 ** (h - r - 1)) dfs(root.right, r + 1, c + 2 ** (h - r - 1)) h = height(root) m, n = h + 1, 2 ** (h + 1) - 1 ans = [[""] * n for _ in range(m)] dfs(root, 0, (n - 1) // 2) return ans -
/** * Definition for a binary tree node. * type TreeNode struct { * Val int * Left *TreeNode * Right *TreeNode * } */ func printTree(root *TreeNode) [][]string { var height func(root *TreeNode) int height = func(root *TreeNode) int { if root == nil { return -1 } return 1 + max(height(root.Left), height(root.Right)) } h := height(root) m, n := h+1, (1<<(h+1))-1 ans := make([][]string, m) for i := range ans { ans[i] = make([]string, n) for j := range ans[i] { ans[i][j] = "" } } var dfs func(root *TreeNode, r, c int) dfs = func(root *TreeNode, r, c int) { if root == nil { return } ans[r][c] = strconv.Itoa(root.Val) dfs(root.Left, r+1, c-int(math.Pow(float64(2), float64(h-r-1)))) dfs(root.Right, r+1, c+int(math.Pow(float64(2), float64(h-r-1)))) } dfs(root, 0, (n-1)/2) return ans } -
/** * Definition for a binary tree node. * class TreeNode { * val: number * left: TreeNode | null * right: TreeNode | null * constructor(val?: number, left?: TreeNode | null, right?: TreeNode | null) { * this.val = (val===undefined ? 0 : val) * this.left = (left===undefined ? null : left) * this.right = (right===undefined ? null : right) * } * } */ function printTree(root: TreeNode | null): string[][] { const getHeight = (root: TreeNode | null, h: number) => { if (root == null) { return h - 1; } return Math.max(getHeight(root.left, h + 1), getHeight(root.right, h + 1)); }; const height = getHeight(root, 0); const m = height + 1; const n = 2 ** (height + 1) - 1; const res: string[][] = Array.from({ length: m }, () => new Array(n).fill('')); const dfs = (root: TreeNode | null, i: number, j: number) => { if (root === null) { return; } const { val, left, right } = root; res[i][j] = val + ''; dfs(left, i + 1, j - 2 ** (height - i - 1)); dfs(right, i + 1, j + 2 ** (height - i - 1)); }; dfs(root, 0, (n - 1) >>> 1); return res; } -
// Definition for a binary tree node. // #[derive(Debug, PartialEq, Eq)] // pub struct TreeNode { // pub val: i32, // pub left: Option<Rc<RefCell<TreeNode>>>, // pub right: Option<Rc<RefCell<TreeNode>>>, // } // // impl TreeNode { // #[inline] // pub fn new(val: i32) -> Self { // TreeNode { // val, // left: None, // right: None // } // } // } use std::rc::Rc; use std::cell::RefCell; impl Solution { fn get_height(root: &Option<Rc<RefCell<TreeNode>>>, h: u32) -> u32 { if let Some(node) = root { let node = node.borrow(); return Self::get_height(&node.left, h + 1).max(Self::get_height(&node.right, h + 1)); } h - 1 } fn dfs( root: &Option<Rc<RefCell<TreeNode>>>, i: usize, j: usize, res: &mut Vec<Vec<String>>, height: u32 ) { if root.is_none() { return; } let node = root.as_ref().unwrap().borrow(); res[i][j] = node.val.to_string(); Self::dfs(&node.left, i + 1, j - (2usize).pow(height - (i as u32) - 1), res, height); Self::dfs(&node.right, i + 1, j + (2usize).pow(height - (i as u32) - 1), res, height); } pub fn print_tree(root: Option<Rc<RefCell<TreeNode>>>) -> Vec<Vec<String>> { let height = Self::get_height(&root, 0); let m = (height + 1) as usize; let n = (2usize).pow(height + 1) - 1; let mut res = vec![vec![String::new(); n]; m]; Self::dfs(&root, 0, (n - 1) >> 1, &mut res, height); res } }