Welcome to Subscribe On Youtube
235. Lowest Common Ancestor of a Binary Search Tree
Description
Given a binary search tree (BST), find the lowest common ancestor (LCA) node of two given nodes in the BST.
According to the definition of LCA on Wikipedia: “The lowest common ancestor is defined between two nodes p and q as the lowest node in T that has both p and q as descendants (where we allow a node to be a descendant of itself).”
Example 1:
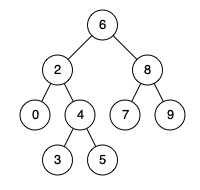
Input: root = [6,2,8,0,4,7,9,null,null,3,5], p = 2, q = 8 Output: 6 Explanation: The LCA of nodes 2 and 8 is 6.
Example 2:

Input: root = [6,2,8,0,4,7,9,null,null,3,5], p = 2, q = 4 Output: 2 Explanation: The LCA of nodes 2 and 4 is 2, since a node can be a descendant of itself according to the LCA definition.
Example 3:
Input: root = [2,1], p = 2, q = 1 Output: 2
Constraints:
- The number of nodes in the tree is in the range
[2, 105]. -109 <= Node.val <= 109- All
Node.valare unique. p != qpandqwill exist in the BST.
Solutions
If the value of the root node is greater than the larger value between p and q, indicating that both p and q are in the left subtree, then we enter the left child node of the root node to continue recursion,
If the root node is less than the smaller value between p and q, indicating that both p and q are in the right subtree, then we enter the right child node of the root node to continue recursion,
If neither, it means that the current root node is the smallest common parent node and return directly.
-
/** * Definition for a binary tree node. * public class TreeNode { * int val; * TreeNode left; * TreeNode right; * TreeNode(int x) { val = x; } * } */ class Solution { public TreeNode lowestCommonAncestor(TreeNode root, TreeNode p, TreeNode q) { while (true) { if (root.val < Math.min(p.val, q.val)) { root = root.right; } else if (root.val > Math.max(p.val, q.val)) { root = root.left; } else { return root; } } } } -
/** * Definition for a binary tree node. * struct TreeNode { * int val; * TreeNode *left; * TreeNode *right; * TreeNode(int x) : val(x), left(NULL), right(NULL) {} * }; */ class Solution { public: TreeNode* lowestCommonAncestor(TreeNode* root, TreeNode* p, TreeNode* q) { while (1) { if (root->val < min(p->val, q->val)) { root = root->right; } else if (root->val > max(p->val, q->val)) { root = root->left; } else { return root; } } } }; -
# Definition for a binary tree node. # class TreeNode: # def __init__(self, x): # self.val = x # self.left = None # self.right = None class Solution: def lowestCommonAncestor( self, root: 'TreeNode', p: 'TreeNode', q: 'TreeNode' ) -> 'TreeNode': while 1: if root.val < min(p.val, q.val): # no =, so root is p or q is in else block root = root.right elif root.val > max(p.val, q.val): root = root.left else: return root ############ class Solution: def lowestCommonAncestor(self, root, p, q): if root is None: return root if root.val > max(p.val, q.val): return self.lowestCommonAncestor(root.left, p, q) elif root.val < min(p.val, q.val): return self.lowestCommonAncestor(root.right, p, q) else: return root ############ class Solution(object): def lowestCommonAncestor(self, root, p, q): """ :type root: TreeNode :type p: TreeNode :type q: TreeNode :rtype: TreeNode """ a, b = sorted([p.val, q.val]) while not a <= root.val <= b: if a > root.val: root = root.right else: root = root.left return root -
/** * Definition for a binary tree node. * type TreeNode struct { * Val int * Left *TreeNode * Right *TreeNode * } */ func lowestCommonAncestor(root, p, q *TreeNode) *TreeNode { for { if root.Val < p.Val && root.Val < q.Val { root = root.Right } else if root.Val > p.Val && root.Val > q.Val { root = root.Left } else { return root } } } -
/** * Definition for a binary tree node. * class TreeNode { * val: number * left: TreeNode | null * right: TreeNode | null * constructor(val?: number, left?: TreeNode | null, right?: TreeNode | null) { * this.val = (val===undefined ? 0 : val) * this.left = (left===undefined ? null : left) * this.right = (right===undefined ? null : right) * } * } */ function lowestCommonAncestor( root: TreeNode | null, p: TreeNode | null, q: TreeNode | null, ): TreeNode | null { while (root) { if (root.val > p.val && root.val > q.val) { root = root.left; } else if (root.val < p.val && root.val < q.val) { root = root.right; } else { return root; } } }