Welcome to Subscribe On Youtube

Formatted question description: https://leetcode.ca/all/2174.html

2174. Remove All Ones With Row and Column Flips II

Description

You are given a 0-indexed m x n binary matrix grid.

In one operation, you can choose any i and j that meet the following conditions:

• 0 <= i < m
• 0 <= j < n
• grid[i][j] == 1

and change the values of all cells in row i and column j to zero.

Return the minimum number of operations needed to remove all 1's from grid.

 

Example 1:

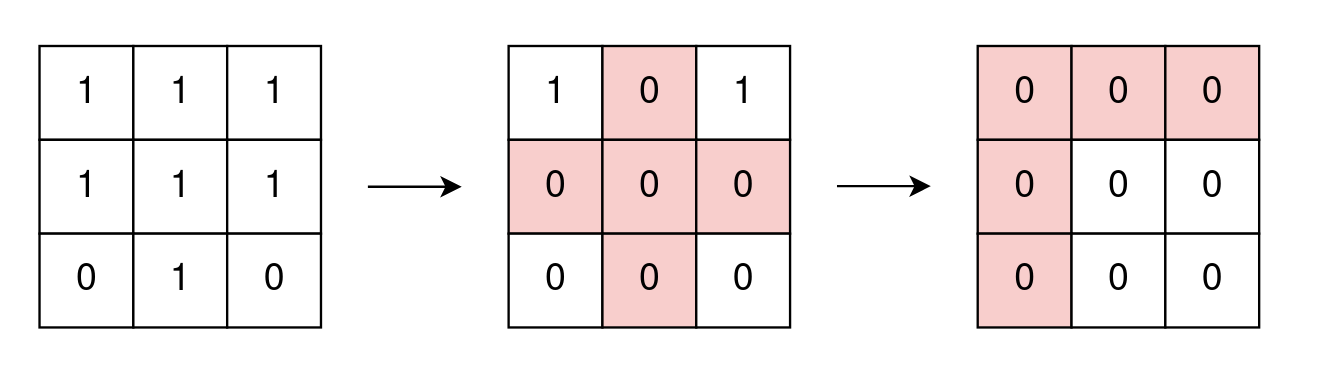
Input: grid = [[1,1,1],[1,1,1],[0,1,0]]
Output: 2
Explanation:
In the first operation, change all cell values of row 1 and column 1 to zero.
In the second operation, change all cell values of row 0 and column 0 to zero.

Example 2:

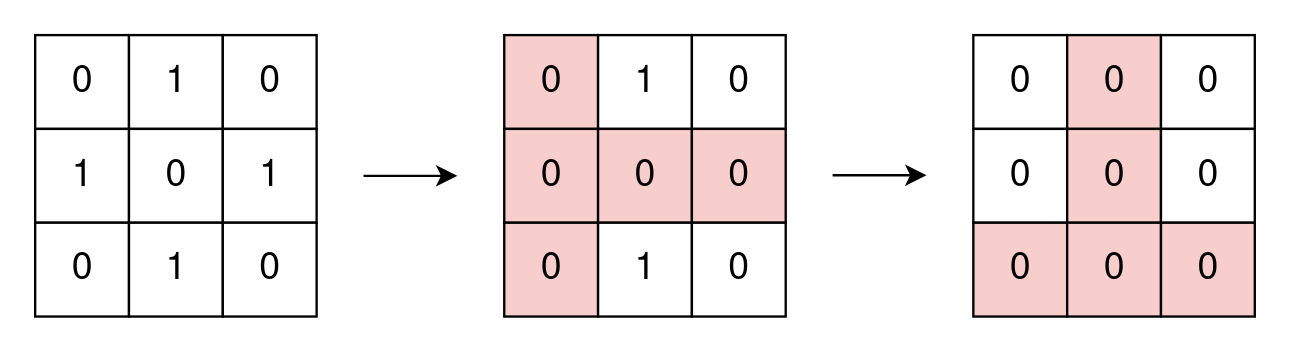
Input: grid = [[0,1,0],[1,0,1],[0,1,0]]
Output: 2
Explanation:
In the first operation, change all cell values of row 1 and column 0 to zero.
In the second operation, change all cell values of row 2 and column 1 to zero.
Note that we cannot perform an operation using row 1 and column 1 because grid[1][1] != 1.

Example 3:

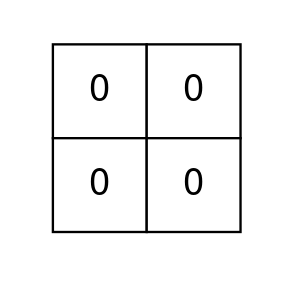
Input: grid = [[0,0],[0,0]]
Output: 0
Explanation:
There are no 1's to remove so return 0.

 

Constraints:

• m == grid.length
• n == grid[i].length
• 1 <= m, n <= 15
• 1 <= m * n <= 15
• grid[i][j] is either 0 or 1.

Solutions

• Java
• C++
• Python
• Go

• class Solution {
      public int removeOnes(int[][] grid) {
          int m = grid.length, n = grid[0].length;
          int state = 0;
          for (int i = 0; i < m; ++i) {
              for (int j = 0; j < n; ++j) {
                  if (grid[i][j] == 1) {
                      state |= 1 << (i * n + j);
                  }
              }
          }
          Deque<Integer> q = new ArrayDeque<>();
          q.offer(state);
          Set<Integer> vis = new HashSet<>();
          vis.add(state);
          int ans = 0;
          while (!q.isEmpty()) {
              for (int k = q.size(); k > 0; --k) {
                  state = q.poll();
                  if (state == 0) {
                      return ans;
                  }
                  for (int i = 0; i < m; ++i) {
                      for (int j = 0; j < n; ++j) {
                          if (grid[i][j] == 0) {
                              continue;
                          }
                          int nxt = state;
                          for (int r = 0; r < m; ++r) {
                              nxt &= ~(1 << (r * n + j));
                          }
                          for (int c = 0; c < n; ++c) {
                              nxt &= ~(1 << (i * n + c));
                          }
                          if (!vis.contains(nxt)) {
                              vis.add(nxt);
                              q.offer(nxt);
                          }
                      }
                  }
              }
              ++ans;
          }
          return -1;
      }
  }
  

• class Solution {
  public:
      int removeOnes(vector<vector<int>>& grid) {
          int m = grid.size(), n = grid[0].size();
          int state = 0;
          for (int i = 0; i < m; ++i)
              for (int j = 0; j < n; ++j)
                  if (grid[i][j])
                      state |= (1 << (i * n + j));
          queue<int> q{ {state} };
          unordered_set<int> vis{ {state} };
          int ans = 0;
          while (!q.empty()) {
              for (int k = q.size(); k > 0; --k) {
                  state = q.front();
                  q.pop();
                  if (state == 0) return ans;
                  for (int i = 0; i < m; ++i) {
                      for (int j = 0; j < n; ++j) {
                          if (grid[i][j] == 0) continue;
                          int nxt = state;
                          for (int r = 0; r < m; ++r) nxt &= ~(1 << (r * n + j));
                          for (int c = 0; c < n; ++c) nxt &= ~(1 << (i * n + c));
                          if (!vis.count(nxt)) {
                              vis.insert(nxt);
                              q.push(nxt);
                          }
                      }
                  }
              }
              ++ans;
          }
          return -1;
      }
  };
  

• class Solution:
      def removeOnes(self, grid: List[List[int]]) -> int:
          m, n = len(grid), len(grid[0])
          state = sum(1 << (i * n + j) for i in range(m) for j in range(n) if grid[i][j])
          q = deque([state])
          vis = {state}
          ans = 0
          while q:
              for _ in range(len(q)):
                  state = q.popleft()
                  if state == 0:
                      return ans
                  for i in range(m):
                      for j in range(n):
                          if grid[i][j] == 0:
                              continue
                          nxt = state
                          for r in range(m):
                              nxt &= ~(1 << (r * n + j))
                          for c in range(n):
                              nxt &= ~(1 << (i * n + c))
                          if nxt not in vis:
                              vis.add(nxt)
                              q.append(nxt)
              ans += 1
          return -1
  
  

• func removeOnes(grid [][]int) int {
  	m, n := len(grid), len(grid[0])
  	state := 0
  	for i, row := range grid {
  		for j, v := range row {
  			if v == 1 {
  				state |= 1 << (i*n + j)
  			}
  		}
  	}
  	q := []int{state}
  	vis := map[int]bool{state: true}
  	ans := 0
  	for len(q) > 0 {
  		for k := len(q); k > 0; k-- {
  			state = q[0]
  			if state == 0 {
  				return ans
  			}
  			q = q[1:]
  			for i, row := range grid {
  				for j, v := range row {
  					if v == 0 {
  						continue
  					}
  					nxt := state
  					for r := 0; r < m; r++ {
  						nxt &= ^(1 << (r*n + j))
  					}
  					for c := 0; c < n; c++ {
  						nxt &= ^(1 << (i*n + c))
  					}
  					if !vis[nxt] {
  						vis[nxt] = true
  						q = append(q, nxt)
  					}
  				}
  			}
  		}
  		ans++
  	}
  	return -1
  }
  

All Problems

All Solutions