A binary expression tree is a kind of binary
tree used to represent arithmetic expressions. Each node of a binary expression tree has
either zero or two children. Leaf nodes (nodes with 0 children) correspond to operands
(numbers), and internal nodes (nodes with 2 children) correspond to the operators '+'
(addition), '-' (subtraction), '*' (multiplication), and
'/' (division).
For each internal node with operator o, the infix
expression that it represents is (A o B), where
A is the expression the left subtree represents and B is
the expression the right subtree represents.
You are given a string s, an infix expression
containing operands, the operators described above, and parentheses '('
and ')'.
Return the binary expression tree, which its in-order
traversal reproduce s.
Please note that order of operations applies
in s. That is, expressions in parentheses are
evaluated first, and multiplication and division happen before addition and
subtraction.
Example 1:
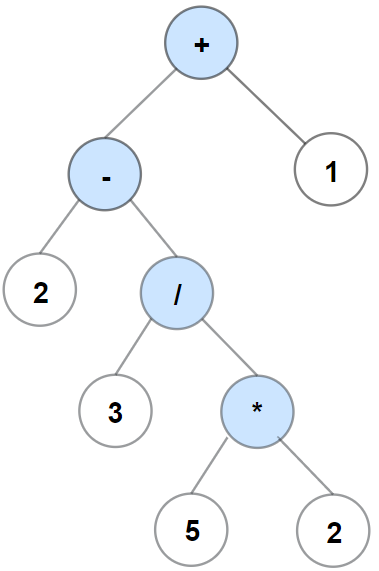
Input: s = "2-3/(5*2)+1" Output: [+,-,1,2,/,null,null,null,null,3,*,null,null,5,2]
Example 2:
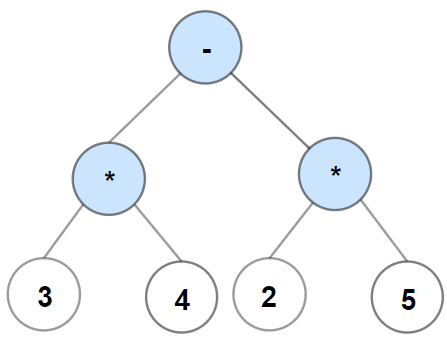
Input: s = "3*4-2*5" Output: [-,*,*,3,4,2,5]
Example 3:
Input: s = "1+2+3+4+5" Output: [+,+,5,+,4,null,null,+,3,null,null,1,2]
Constraints:
1 <= s.length <= 105sconsists of digits and the characters'+','-','*','/','(', and')'.- Operands in
sare exactly 1 digit. - It is guaranteed that
sis a valid expression.